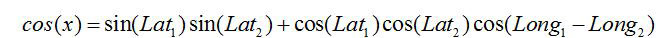Challenge 6 – As the Crow Flies on a Spherical Planet
In geography, we note the positions of features on Earth’s surface using latitude and longitude measure, which describe the angular locations on the two-dimensional surface of Earth. But we also want to know how far apart features are in terms of walking, driving or jet-travel distances.
Globe with geodesic path segment
Credit: US Geological Survey – Online Earthquake Glossary: Great circle.
The shortest distance between any two points on the surface of a sphere is called the Great Circle, a part of which is shown in the diagram as a dashed line. This circle is concentric with the center of the sphere. All lines of constant longitude are Great Circles, but only the equatorial circle of the equator is a Great Circle of latitude. All other latitude circles are not Great Circles because they do not include the center of Earth as a point on the intersecting plane.
Once two points on the surface of Earth have been identified and the Great Circle drawn through them, we can draw an angle with a vertex at the center of Earth, with its two segments intersecting the Great Circle at the two points. The size of this angle, X, can be found from the formula
where the geographic latitude and longitude of the two points are (Lat1, Long1) and (Lat2, Long2). Because the circumference of every Great Circle is just the equatorial circumference of Earth, 40000 km, we can find the distance between the two points from the proportion D = (X/360) 40000 km.
Example: For Carbondale (37.56, 89.06) and Hopkinsville (36.87, 87.47) we have:
cos(X) = sin(37.56)sin(36.87) + cos(37.56)cos(36.87)cos(89.06-87.47)
cos(X) = 0.9997
so X = 1.4 degrees, and the distance is then 40000 x (1.4/360) = 155 km.
Problem 1 - The path of the August 21, 2017 total solar eclipse reaches the Pacific Coast of Oregon at latitude 44.8439 North and longitude 124.051West, and travels across the continental United States until it exits South Carolina on the Atlantic Coast at latitude 33.0354 North and longitude 79.4869 West. What is the total length of the path of the eclipse across the continental United States?
Problem 2 - An eclipse watcher from Washington, DC wants to travel to the closest spot on the East Coast where the total solar eclipse can be viewed. If his home is near latitude 38.9 North and 77.05 West and the closest point on the eclipse track is near Columbia, South Carolina at latitude 34.00 North and longitude 81.05 West, what is the straight-line, crow-flies distance to get to the eclipse site from Washington, DC?